Search for answers or browse our Knowledge Base.
Guides | Models | Validation | Book
-
Guides
-
-
- New Tools in AN-SOF: Selecting and Editing Wires in Bulk
- How to Speed Up Simulations in AN-SOF: Tips for Faster Results
- Enhancing Antenna Design Flexibility: Project Merging in AN-SOF
- AN-SOF Antenna Simulation Best Practices: Checking and Correcting Model Errors
- How to Adjust the Radiation Pattern Reference Point for Better Visualization
- H-Field Option in Preferences
-
- Can AI Design Antennas? Lessons from a 3-Iteration Yagi-Uda Experiment
- Modeling Common-Mode Currents in Coaxial Cables: A Hybrid Approach
- Beyond Analytical Formulas: Accurate Coil Inductance Calculation with AN-SOF
- Complete Workflow: Modeling, Feeding, and Tuning a 20m Band Dipole Antenna
- DIY Helix High Gain Directional Antenna: From Simulation to 3D Printing
- Design Guidelines for Skeleton Slot Antennas: A Simulation-Driven Approach
- Simplified Modeling for Microstrip Antennas on Ungrounded Dielectric Substrates: Accuracy Meets Simplicity
- Fast Modeling of a Monopole Supported by a Broadcast Tower
- Linking Log-Periodic Antenna Elements Using Transmission Lines
- AN-SOF Mastery: Adding Elevated Radials Quickly
- An Efficient Approach to Simulating Radiating Towers for Broadcasting Applications
- RF Techniques: Implicit Modeling and Equivalent Circuits for Baluns
-
- Understanding the Antenna Near Field: Key Concepts Every Ham Radio Operator Should Know
- Evaluating EMF Compliance - Part 1: A Guide to Far-Field RF Exposure Assessments
- Evaluating EMF Compliance - Part 2: Using Near-Field Calculations to Determine Exclusion Zones
- Wave Matching Coefficient: Defining the Practical Near-Far Field Boundary
- AN-SOF Data Export: A Guide to Streamlining Your Workflow
- Front-to-Rear and Front-to-Back Ratios: Applying Key Antenna Directivity Metrics
- Export Radiation Patterns to MSI Planet
- Export Radiation Patterns to Radio Mobile
- Scilab Script for Plotting Level Curves
- Adjusting the Color Bar in AN-3D Pattern
-
-
-
- Introducing AN-SOF 10.5 – Smarter Tools, Faster Workflow, Greater Precision
- Introducing the AN-SOF Engine: Power, Speed, and Flexibility for Antenna Simulation
- What’s New in AN-SOF 10? Smarter Tools for RF Professionals and Antenna Enthusiasts
- To Our Valued AN-SOF Customers and Users: Reflections, Milestones, and Future Plans
- AN-SOF 9.50 Release: Streamlining Polarization, Geometry, and EMF Calculations
- AN-SOF 9: Taking Antenna Design Further with New Feeder and Tuner Calculators
- AN-SOF Antenna Simulation Software - Version 8.90 Release Notes
- AN-SOF 8.70: Enhancing Your Antenna Design Journey
- Introducing AN-SOF 8.50: Enhanced Antenna Design & Simulation Software
- Get Ready for the Next Level of Antenna Design: AN-SOF 8.50 is Coming Soon!
- Explore the Cutting-Edge World of AN-SOF Antenna Simulation Software!
- Upgrade to AN-SOF 8.20 - Unleash Your Potential
- AN-SOF 8: Elevating Antenna Simulation to the Next Level
- New Release: AN-SOF 7.90
- AN-SOF 7.80 is ready!
- New AN-SOF User Guide
- New Release: AN-SOF 7.50
- AN-SOF 7.20 is ready!
- New Release :: AN-SOF 7.10 ::
- AN-SOF 7.0 is Here!
- New Release :: AN-SOF 6.40 ::
- New Release :: AN-SOF 6.20 ::
- Show All Articles (7) Collapse Articles
-
-
- Types of Wires
- Wire Attributes
- Wire Materials
- Enabling/Disabling Resistivity
- Enabling/Disabling Coating
- Cross-Section Equivalent Radius
- Exporting Wires
-
-
Models
-
- Download Examples
- Explore 5 Antenna Models with Less Than 50 Segments in AN-SOF Trial Version
- Modeling a Center-Fed Cylindrical Antenna with AN-SOF
- Modeling a Circular Loop Antenna in AN-SOF: A Step-by-Step Guide
- Monopole Antennas Over Imperfect Ground: Modeling and Analysis with AN-SOF
- Modeling Helix Antennas in Axial Radiation Mode Using AN-SOF
- Step-by-Step: Modeling Basic Yagi-Uda Arrays for Beginners
- A Transmission Line
- An RLC Circuit
-
- Pi Day Special: A Short Dipole with Radiation Resistance of 3.14 Ohms
- Modeling a Super J-Pole: A Look Inside a 5-Element Collinear Antenna
- The 5-in-1 J-Pole Antenna Solution for Multiband Communications
- Simulating a Multiband Omnidirectional Dipole Antenna Design
- The Loop on Ground (LoG) Antenna: A Compact Solution for Directional Reception
- Precision Simulations with AN-SOF for Magnetic Loop Antennas
- Advantages of AN-SOF for Simulating 433 MHz Spring Helical Antennas for ISM & LoRa Applications
- Understanding the Folded Dipole: Structure, Impedance, and Simulation
- Radio Mast Above Wire Screen
- Experimenting with Half-Wave Square Loops: Simulation and Practical Insights
- Radar Cross Section and Reception Characteristics of a Passive Loop Antenna: A Simulation Study
- Monopole Above Earth Ground
- Design and Simulation of Short Top-Loaded Monopole Antennas for LF and MF Bands
- Half-Wave Dipole
- Dipole Antenna
-
- Efficient NOAA Satellite Signal Reception with the Quadrifilar Helix Antenna
- Inverted V Antenna
- Boosting Performance with Dual V Antennas: A Practical Design and Simulation
- Helical Antenna with Grid Reflector
- Helical Antenna with PEC Reflector
- 7-Element Yagi-Uda
- 5-Element Yagi-Uda
- 3-Element Yagi-Uda
-
- Exploring an HF Log-Periodic Sawtooth Array: Insights from Geometry to Simulation
- The Lazy-H Antenna: A 10-Meter Band Design Guide
- Extended Double Zepp (EDZ): A Phased Array Solution for Directional Antenna Applications
- Transmission Line Feeding in Antenna Design: Exploring the Four-Square Array
- Enhancing VHF Performance: The Dual Reflector Moxon Antenna for 145 MHz
- Building a Compact High-Performance UHF Array with AN-SOF: A 4-Element Biquad Design
- Building a Beam: Modeling a 5-Element 2m Band Quad Array
- A Closer Look at the HF Skeleton Slot Antenna
- The 17m Band 2-Element Delta Loop Beam: A Compact, High-Gain Antenna for DX Enthusiasts
- The Moxon-Yagi Dual-Band VHF/UHF Antenna for Superior Satellite Link Performance
- Broadside Dipole Array
- Log-Periodic Dipole Array
- Broadband Directional Antenna
- Log-Periodic Christmas Tree
-
- Nelder-Mead Optimization for Antenna Design Using the AN-SOF Engine and Scilab
- Evolving Better Antennas: A Genetic Algorithm Optimizer Using AN-SOF and Scilab
- Building Effective Cost Functions for Antenna Optimization: Weighting, Normalization, and Trade-offs
- Element Spacing Simulation Script for Yagi-Uda Antennas
- Automating 2-Element Quad Array Design: Scripting and Bulk Processing in AN-SOF
-
-
Validation
-
- Simple Dual Band Vertical Dipole for the 2m and 70cm Bands
- Linear Antenna Theory: Historical Approximations and Numerical Validation
- Validation of a Panel RBS Antenna with Dipole Radiators against IEC 62232 Standard
- Validating V Antennas: Directivity Analysis with AN-SOF
- Enhanced Methodology for Monopoles Above Radial Wire Ground Screens
- Validating Dipole Antenna Simulations: A Comparative Study with King-Middleton
- Dipole Gain and Radiation Resistance
- Convergence of the Dipole Input Impedance
-
Book
-
- 1.0 Table of Contents
- 1.1 Maxwell’s Equations and Electromagnetic Radiation
- 1.2 The Isotropic Radiator
- 1.3 Arrays of Point Sources
- 1.4 The Hertzian Dipole
- 1.5 The Short Dipole – FREE SAMPLE
- 1.6 The Half-Wave Dipole
- 1.7 Thin Dipoles of Arbitrary Length
- 1.8 Ground Plane and Image Theory
- 1.9 The Hertzian Monopole
- 1.10 Quarter-Wave and General Monopoles
-
- 2.1 Radiation Pattern Fundamentals
- 2.2 Field Strength and Intensity Plots
- 2.3 Total Radiated Power
- 2.4 Radiation Resistance
- 2.5 Antenna Effective Length
- 2.6 Loss Resistance Modeling
- 2.7 Radiation Efficiency
- 2.8 Directivity and Gain
- 2.9 Beamwidth and Sidelobes
- 2.10 Feedpoint Impedance and Bandwidth
- 2.11 Receiving Mode Operation
- 2.12 Equivalent Circuits for TX/RX
- 2.13 The Reciprocity Principle
- 2.14 Effective Aperture and Gain
- 2.15 The Friis Transmission Equation
-
- 3.1 Cylindrical Wire Antennas
- 3.2 Feedpoint Resistance and Reactance
- 3.3 Calculating Input Impedance
- 3.4 Induced EMF Method
- 3.5 Hallén’s Integral Equation
- 3.6 Pocklington’s Integral Equation
- 3.7 Equivalent Radius for Non-Circular Wires
- 3.8 Impedance of Short Dipoles and Monopoles
- 3.9 Top-Loading for Short Monopoles
- 3.10 Mutual Impedance Between Wires
-
Gain of Helix in Normal Mode
Helical Geometry
The current distribution in a helical antenna of arbitrary size cannot be described in terms of simple series or elementary functions, so most of the available data has been obtained experimentally. The helical antenna was invented by Prof. John Krauss who has investigated its performance as a function of geometry extensively. Refer to “Antennas” by John D. Krauss, 2nd Edition, McGraw-Hill, 1988.
The helix has basically two modes of operation,
- The normal mode or broadside mode where the maximum radiation occurs in the plane normal to the helix axis.
- The axial mode or end-fire mode where the maximum radiation occurs along the helix axis.
The normal mode is obtained in helices that are small compared to the wavelength, therefore their behavior is similar to that of a small loop > with the same number of turns as the helix and with a uniform current distribution. This can be considered as a limiting case and the simulation results can be compared with theory. When the helix size is comparable to the wavelength, the current distribution can no longer be considered uniform and the simulation results will be only compared with measurements.
The helix geometry is usually described in terms of its circumference normalized to the wavelength, C/λ (C = 2πR, R being the helix radius), number of turns, N, and pitch angle, α, defined as
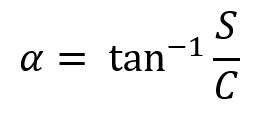
S being the pitch or spacing between turns. The starting point and the axis direction of the helix can also be set in AN-SOF, as Fig. 1 shows.
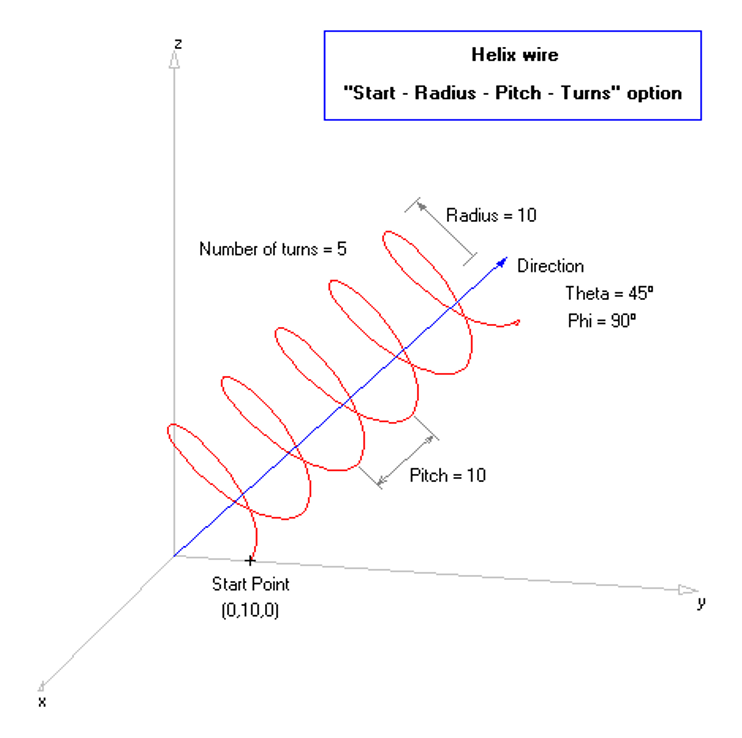
In the simulations that follow the helix axis will be the z-axis. Also, a ground plane will be placed at z = 0, so the starting point of the helix will be (0,0,Zs), with Zs being small compared to the wavelength. The helix will be connected to the ground plane by means of a short wire having one segment, where a voltage source will be placed.
Normal Mode
For the normal mode of operation, an small helix having a circumference C/λ = 0.01, 5 turns and a variable pitch will be simulated. The pitch varies between 0.001λ and 0.01λ. For these small dimensions, the theoretical prediction is that the radiation pattern must be doughnut-shaped as that of a small loop, with a null along the helix axis. Moreover, the gain in the presence of a perfect ground plane must be 3 (dimensionless) or 4.77 dBi.
Fig. 2 shows the computed 3D radiation patterns of the small helix for a pitch of 0.001λ (left) and 0.01λ (right). It can be seen that the results correspond to the theoretical predictions. The small departure from 4.77 dBi obtained for the largest pitch must be interpreted as a deviation from the theoretical limiting case of an infinitely small helix. This behavior can be appreciated if we plot the peak gain as a function of the pitch, as in Fig. 3, where we can see that the computed gain tends to 4.77 dBi as the pitch tends to zero.
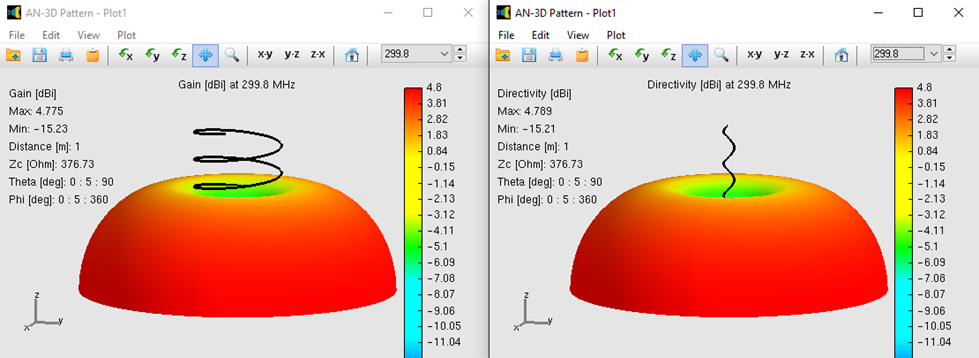
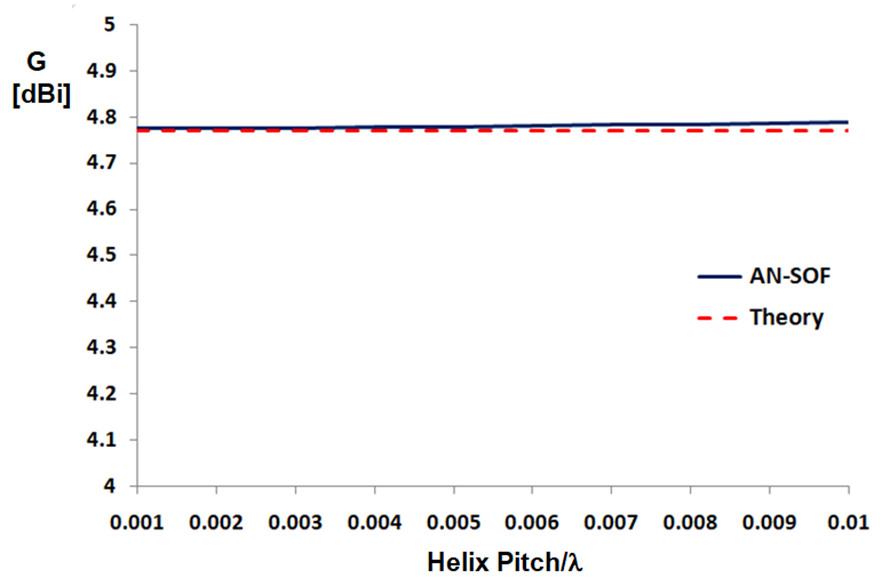
This result demonstrates the ability of AN-SOF to simulate curved structures with a small size compared to the wavelength.
