Power Budget

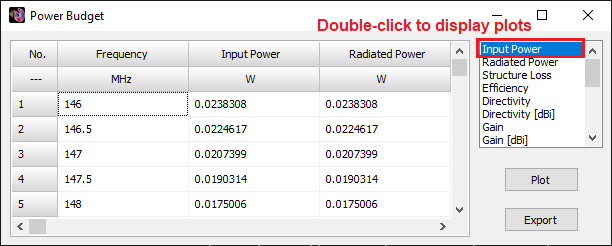
To access the Power Budget dialog box (see Fig. 1), navigate to Results > Power Budget/RCS in the main menu. When discrete sources are used for excitation, the following list of parameters versus frequency is displayed:

Parameters

• Input Power: Total input power provided by the discrete sources in the structure.

• Radiated Power: Total radiated power from the structure.

• Structure Loss: Total consumed power, representing ohmic losses in the structure.

• Efficiency: Radiated power-to-input power ratio, representing the radiation efficiency. For a lossless structure, this value is 100%.

• Directivity: Peak directivity, displayed both as a dimensionless value and in decibels (dBi) relative to an isotropic source.

• Gain: Peak gain, displayed both as a dimensionless value and in decibels (dBi) relative to an isotropic source.

• Av. EIRP (Effective Isotropic Radiated Power): Time-averaged EIRP in Watts and dBW. This value accounts for the duty cycle of the selected transmit mode in the Tuner tab and the Time Transmitting percentage.

• Peak EIRP (Effective Isotropic Radiated Power): Peak EIRP in Watts and dBW, calculated directly from the Peak Envelope Power (PEP) without considering the duty cycle or time transmitting percentage.

• Av. Power Density: Average power density, calculated by averaging the power density over all directions in space.

• Peak Power Density: Maximum value of the radiated power density.

• Theta (max) and Phi (max): The zenith and azimuth angles, respectively, in the direction of maximum radiation, corresponding to the peak power density.

• F/R H and F/B H: Front-to-rear and front-to-back ratios, respectively, in a horizontal slice of the radiation pattern given by Theta = Theta (max).

• F/R V and F/B V: Front-to-rear and front-to-back ratios, respectively, in a vertical slice of the radiation pattern given by Phi = Phi (max).

Error and Average Gain Test (AGT)

• Error: Represents the error in the power balance of the system. A necessary (but not sufficient) condition for a valid model is that the input power must equal the sum of the radiated and lost powers. The error is calculated as:

$\displaystyle \text{Error %} = 100 \times \frac{\text{Input Power} \,-\, \text{Lost Power} \,-\, \text{Radiated Power}}{\text{Input Power} \,-\, \text{Lost Power}}$

• Average Gain Test (AGT): A validation metric that should be close to 1 for a valid model. It is calculated as:

$\displaystyle \text{AGT} = \frac{\text{Radiated Power} + \text{Lost Power}}{\text{Input Power}}$

Plotting and Exporting Data

• Select an item from the list in the upper-right corner of the window and click the Plot button to plot the selected item versus frequency.

• Click the Export button to export the list to a CSV file.

Notes

• A power budget error of about ±10% is permissible from the engineering point of view.

• When a real ground plane is used, the Error column shows the percentage of power lost in the ground due to its finite conductivity.

• When a substrate slab is used, this column shows the percentage of power transferred to the dielectric material in the substrate.

• AGT = 1 means that the power balance is exact. An AGT between 0.99 and 1.01 is comparable to achieving an error of ±1%.